Problem 1 (Compute a Moment of Inertia). An ellipsoid is centered at \((0,3,0)\); its axes are parallel to the \(x\)-, \(y\)-, and \(z\)-axes. Its longest axis is along the \(y\)-direction, ranging from the origin to \((0,6,0)\); in the plane perpendicular to the \(y\)-axis and going through the center of the ellipsoid at \((0,3,0)\), the cross-section of the ellipsoid is a circular disk with radius \(1\). The body has mass density \(1\).

Compute the moment of inertia with respect to the \(x\)-axis.

Problem 2 (Area of a Surface). The surface \(S\) is defined by the parametrization

where \(u\) ranges from \(0\) to \(1\) and \(t\) ranges from \(-\pi/2\) to \(\pi/2\).

Find the area of \(S\).

Problem 3 (Volume via Triple Integral). A domain \(D\) in \(3\) dimensions is defined as follows: it is the region inside the paraboloid \(z=x^2+y^2\) that lies above the plane \(z=1\) and below the plane \(x+2z=6\). Sketch the top view, the front view and the side view (i.e. looking "straight down" the \(z\)-axis, "straight down" the \(x\)-axis, and "straight down" the \(y\)-axis) of this domain, and set up the integral(s) to compute the volume (no need to compute the outcome).

Problem 4 (Volume via Double Integral). Compute the volume bounded by the two paraboloids \(z=7x^2+y^2-1\) and \(z=15-x^2-7y^2\).

Problem 5 (Compute Surface Area). Compute the following surface areas:

(a) The part on the sphere \(x^2+y^2+z^2=a^2\) contained within cylinder \(x^2+y^2=ax\);

(b) The part on the cone \(z=\sqrt{x^2+y^2}\) contained within the cylinder \(z^2=2x\);

(c) The surface area of the volume obtained by intersecting the two cylinders \(x^2+y^2=R^2\) and \(x^2+z^2=R^2\).

Problem 6 (Coordinate Transformation and Jacobian). Consider the coordinate transformation, defined for all \((x,y,z)\) in \(\mathbb{R}^3\),

where \(r\) ranges over all the non-negative real numbers, \(\alpha\) ranges from \(0\) to \(\pi\), and \(\beta\) from \(0\) to \(2\pi\).

Determine the volume of the region defined by \(1\leq r\leq 2\).

Problem 7 (Triple Integral). A solid in the first octant is bounded by the planes \(y=0\) and \(z=0\) and by the surfaces \(z=4-x^2\) and \(x=y^2\). Its density function is \(\delta(x,y,z)=3xy\).

(a) Find the mass of the solid;

(b) Find tis center of mass.

Problem 8 (Area of a Curvilinear "Hexagon"). Consider the following region (with boundaries marked by bold lines) enclosed by the lines and curves with equations

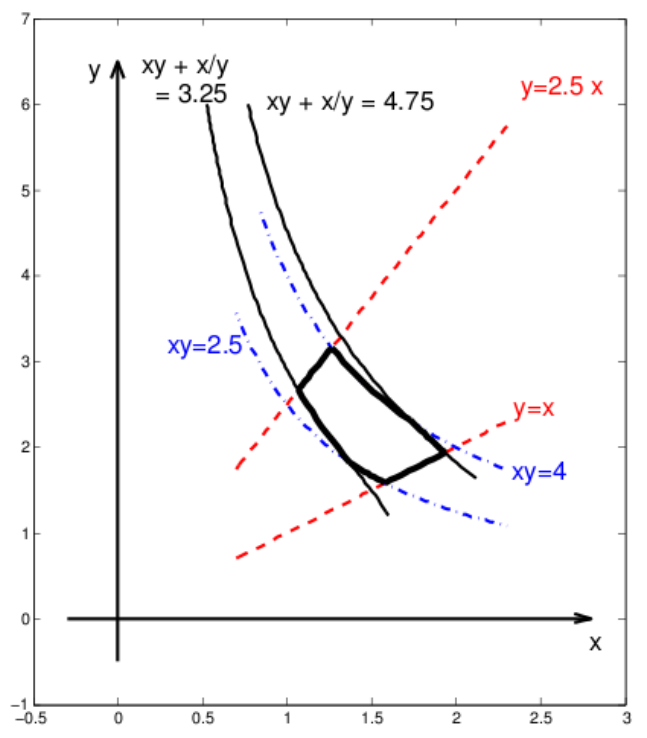
(a) Show that in the new variables \(u=xy, v=x/y\) the region becomes quite simple.

(b) Write the area to be composed as an integral over the variables \(u\) and \(v\).

(c) Compute the integral, and thus the area.

Problem 9. (a) Compute the area of that part of the surface of the cylinder \(x^2+y^2=2ay\) which lies between the \(xy\)-plane and the cone \(y^2=x^2+z^2\).

(b) Compute the area of that part of the surface of the cylinder \(x^2+y^2=2ax\) which lies between the \(xy\)-plane and the cone \(z^2=x^2+y^2\).

Answers

Problem 1. \(44\pi\)

Problem 2. \(\frac{1}{2}e-\frac{3}{2e}\)

Problem 3. \(\int_0^1\!\!\!\int_0^{2\pi}\!\!\!\int_1^{\frac{6-r\cos\theta}{2}}\,dz\,d\theta\,rdr+\int_0^{2\pi}\!\!\!\int_1^{\frac{1}{4}\left[\sqrt{48+\cos^2\theta}-\cos\theta\right]}\!\!\!\int_{r^2}^{\frac{6-r\cos\theta}{2}}\,dz\,rdr\,d\theta\)

Problem 4. \(16\pi\)

Problem 5. (a) \(2a^2\left(\pi-2\right)\quad\) (b) \(\sqrt{2}\pi\quad\) (c) \(16R^2\)

Problem 6. \(8\pi\left(4\sqrt{5}-\sqrt{2}\right)\)

Problem 7. (a) \(\frac{32}{5}\quad\) (b) \(\left(\frac{5}{4}, \frac{40}{77}\sqrt{2}, \frac{8}{7}\right)\)

Problem 8. (a) \(\frac{13}{4}=3.25\leq u+v\leq 4.75=\frac{19}{4}\), \(\frac{5}{2}=2.5\leq u\leq 4\), \(0.4\leq v\leq 1\)

(b) \(\frac{1}{2v}\quad\) (c) \(\frac{3}{8}\ln \frac{40}{9}+\frac{1}{20}\)

Problem 9. (a) \(\sqrt{2}\pi a^2\quad\) (b) \(8a^2\)